Correcting the eyesight of microscopes
Scientists have discovered a new property of wave propagation, that leads to an all-new way to improve the resolution of virtually all optical technologies, including: microscope lenses, telecommunications, laser-based lithography, biological and astronomical imaging. All these systems transmit information and energy through wave propagation. Researchers at the Center for Soft and Living Matter, within the Institute for Basic Science (IBS, South Korea) have discovered that, if light passes through asymmetric apertures, astigmatism arises and can degrade image resolution. Having identified this previously-unsuspected problem, the researchers showed how to remedy it.
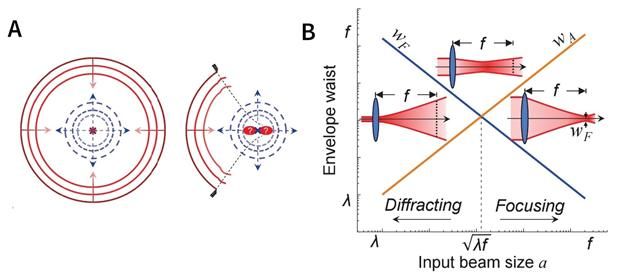
(A) A stone falling in a pond produces full circular waves that are centered on the impact point, and those waves would back propagate onto that same point if time could be reversed. Using this time-reversal argument, if one generates back propagating circular waves from a limited arc, they will not necessarily focus at the center. (B) Representation of how diffraction effects compete with focusing for a beam of different initial size, that is different aperture. Going from left to right on the x-axis, the input beam size (aperture size , orange line) increases. For a large beam, focusing is strong, leading to a small cross section in the focal plane (blue line). If the aperture is reduced, a critical situation is reached (dashed line) where is same as . At the critical point where the two lines cross, focusing and diffraction effects are equal, and energy (red) is best focused between the lens and the initial focal plane, which means that the effective focal plane has shifted towards the lens. Extremely small apertures correspond to a point source and produce diffraction without focusing.
Institute for Basic Science (IBS)
As you read this article, the eye lens focuses light from the screen to the back of the eye. However, if the lens's horizontal and vertical focusing power is different, this text will appear blurred: for example, the vertical and horizontal lines, which form the letter "T" will not be brought in focus together. To avoid this focusing defect, artificial lenses are optimally designed to change the shape of the light wavefronts from planar into perfectly spherical wavefronts, because it is believed that spherical wavefronts necessarily focus at their unique center of curvature. Published in Proceedings of the National Academy of Sciences of the USA (PNAS), this study shows that scientists should re-examine this belief and revisit their design strategies.
Examples of wave propagation are circular waves created by a pebble dropped into a pond. The exact point where the pebble hits the water determines the position and the shape of the waves. If you could go back in time, those circular waves would refocus on the initial impact point precisely, because the information on the point location is not lost during wave propagation. This 2D example can be extended to a 3D situation, where waves are spherical and refocus exactly at the center of the sphere. However, in real life, one generally focuses light from one side, along some direction and not from all directions, and the ideal picture of focusing from a full circle or a full sphere is never exactly relevant.
"A full spherical wave is symmetric and has its focus exactly at the center of the sphere. However, to keep this spherical symmetry, light should propagate from all directions onto the sample. And this virtually never happens. Practically, wavefronts are passed through an aperture that is limited to a portion of a sphere, instead of the full sphere. Consequently, spherical symmetry is broken and information is lost," says Francois Amblard, co-author of the study. In the case of the pond, this would be similar to going back in time to try to refocus a limited wave arc, instead of the full circular waves: these arc waves would not necessarily converge on the same impact point, because information about the center location is partially lost.
The IBS team has explained and given the experimental proof that, as the aperture gets smaller, the focus shifts more and more backwards towards the lens, such that the initial focus is no longer "in focus." As a consequence, if the aperture is not equal in the vertical and horizontal planes, focal shifts will differ between these directions, leading to astigmatism. "Astigmatism can occur even with the most perfect lens if it is used with a non-circular aperture," explains Kai Lou, first author of the study.
The team applied the idea to improve a technique called line-temporal focusing microscopy (LTFM, also named spatiotemporal focusing), which makes use of a naturally asymmetric input beam. As LTFM is a method used to visualize deep biological structures, the researchers tested their focal shift correction strategy with mouse lung tissues. An unprecedented resolution was obtained, that even outperformed a classical technique called point scanning microscopy (PSM).
How does this knowledge help to improve resolution? Even though this effect is very small and can be neglected for ordinary applications, correcting for aperture-induced astigmatism could make a significant difference in delicate systems, like advanced microscopy used to acquire a large volume of images. Understanding that astigmatism is intrinsic to the broken circular symmetry could help design corrections tailored to the aperture shape, especially in fields such as astronomy, telecommunication, or with ultrasounds, where non-circular apertures cannot be avoided.
"In the future, we plan to apply aperture-induced astigmatism to even more complex information transfer technologies," said Steve Granick, co-correspondent author of this study. "Moreover, the study opens avenues to basically improve the design of any equipment handling electromagnetic waves, ultrasounds, or particles beams. For example, it also applies to waves, used with space antennas to focus on satellite or spaceship. We believe it can contribute to design better systems in synthetic microscopic eyesight, telecommunications, and even microwave devices."
Original publication
Other news from the department science
Get the life science industry in your inbox
From now on, don't miss a thing: Our newsletter for biotechnology, pharma and life sciences brings you up to date every Tuesday and Thursday. The latest industry news, product highlights and innovations - compact and easy to understand in your inbox. Researched by us so you don't have to.
